Dyscalculia and Dyslexia
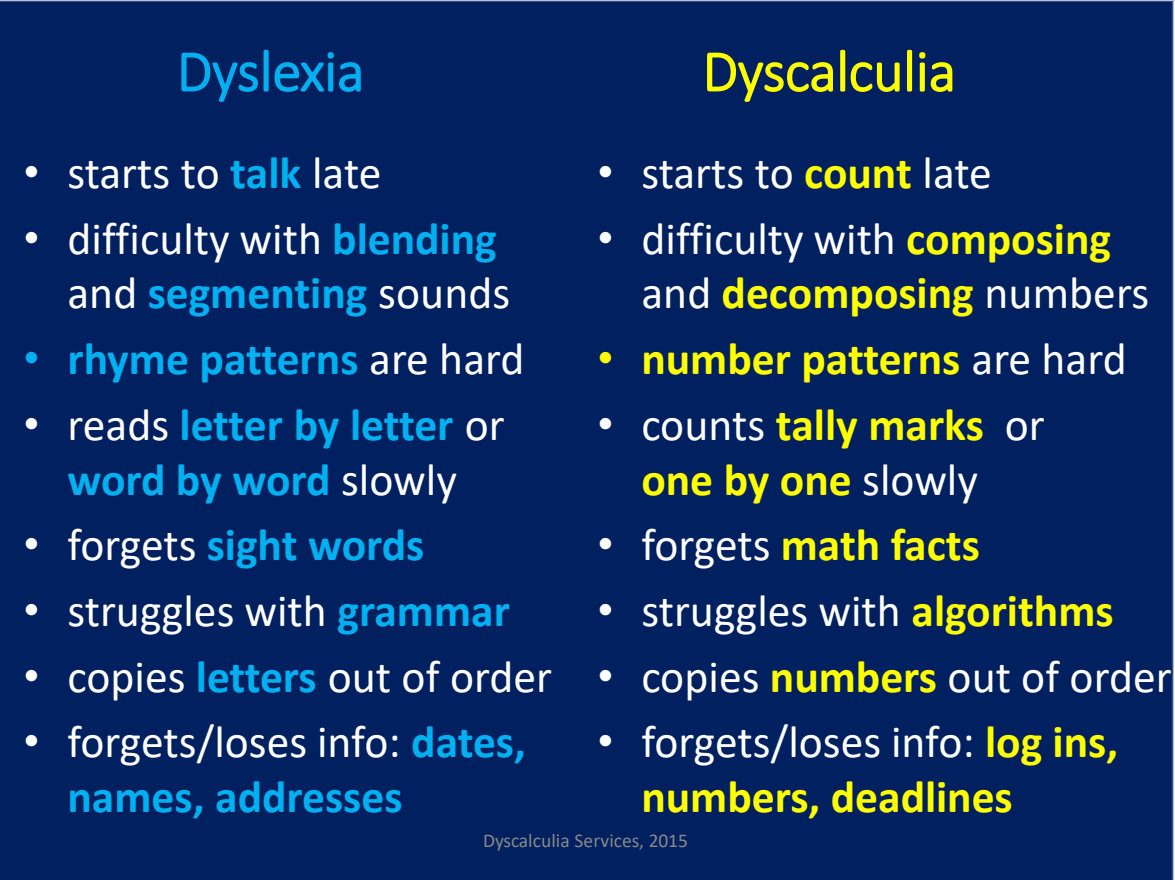
When you know about Dyslexia, you already know a lot about Dyscalculia, here are some of the symptoms compared
How do you determine it is Dyscalculia
This is an important question with implications for the remediation of the condition. At this moment there is no generally agreed definition about what Dyscalculia is but there are now, […]
Dyslexia and Dyscalculia
If you are already familiar with Dyslexia, it is easier to understand the things that happen when you have Dyscalculia. Although they are very different, here is an overview of […]
Is math giving them trouble this year?
The year has started, the first ERB testing has been done, the first report cards are around the corner. We are celebrating Learning Disabilities month, so all reasons to do […]